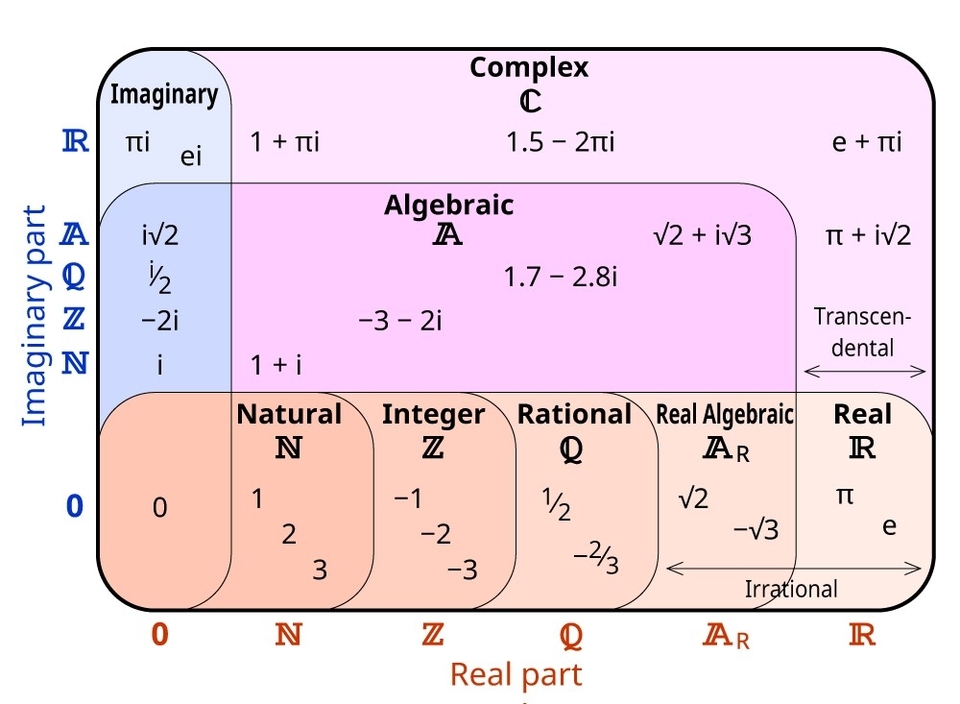
Complex numbers are a fundamental concept in mathematics that extends the real numbers with a specific element denoted `i`, called the imaginary unit. Every complex number can be expressed in the form `a + bi`, where `a` and `b` are real numbers. The real part of a complex number is denoted by `Re(z)` and the imaginary part is denoted by `Im(z)`.
The imaginary unit `i` satisfies the equation `i^2 = -1`. Despite the historical nomenclature “imaginary”, complex numbers are regarded in the mathematical sciences as just as “real” as the real numbers and are fundamental in many aspects of the scientific description of the natural world .
A complex number can be visually represented as a pair of numbers `(a, b)` forming a vector on a diagram called an Argand diagram, representing the complex plane. The real axis is denoted by `Re` and the imaginary axis is denoted by `Im`. The set of complex numbers is denoted by either of the symbols `C` or `?` .
The addition, subtraction, and multiplication of complex numbers can be naturally defined by using the rule `i^2 = -1` combined with the associative, commutative, and distributive laws. Every nonzero complex number has a multiplicative inverse. This makes the complex numbers a field that has the real numbers as a subfield .
The complex numbers also form a real vector space of dimension two, with `{1, i}` as a standard basis. This standard basis makes the complex numbers a Cartesian plane, called the complex plane. This allows a geometric interpretation of the complex numbers and their operations, and conversely expressing in terms of complex numbers some geometric properties and constructions .
Complex numbers allow solutions to all polynomial equations, even those that have no solutions in real numbers. More precisely, the fundamental theorem of algebra asserts that every non-constant polynomial equation with real or complex coefficients has a solution which is a complex number. For example, the equation `x^2 + 1 = 0` has no real solution, since the square of a real number
